Calculate Percentage Uncertainty
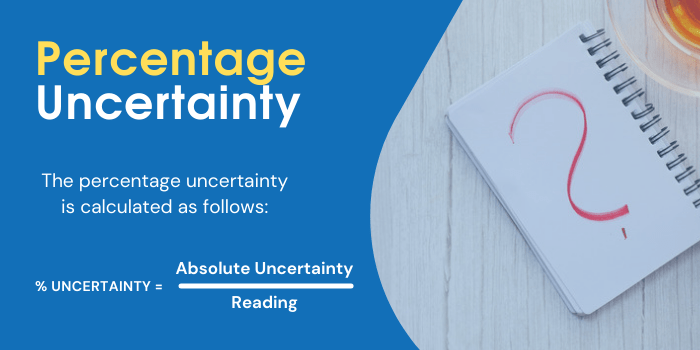
When you measure, you must calculate what amount of ” residuum” or space the measurement results in terms of its range. This space is often referred to as “Relative Discrepancy” or “RAD”. To determine percentage uncertainty, it is necessary to first find out the range that can be measured by the measurement. After that, you should extrapolate the results to determine the amount of the estimate. Also, you can determine the range of expected results and determine the standard deviation. This is the standard deviation used to calculate the distribution of data, or “averages”.
How do you calculate the percentage uncertainty?
The first step is to understand what we’re measuring. When only one measurement is being made like the price of a vehicle’s gross for the month in question, the method to calculate the percentage of uncertainty is fairly easy. There are issues when multiple parameters or an estimator are being used to quantify the measure. In such situations, it is essential to apply an analysis of statistical significance on the response variable in order to determine which estimate is the most accurate.
The standard deviation may be calculated by anybody who has access to a basic library of reference materials. For instance, how do you calculate your standard deviation for two variables randomly? Simply divide the difference in the form of your standard deviation. A smaller gap (less than zero) implies greater uncertainty about the outcome.
Loggers also called “logisticians”, are useful to determine the accuracy of estimates based on measurements. Loggers can be described as statistical models that presume that observations are distributed in a normal manner and are used to calculate their estimations. To calculate the reliability, the degree of deviation should be compared with an average distribution result. The normal range of distribution includes a mean and the standard deviation. Loggers will vary the range in order to calculate a confidence range within the range. The range can then be compared to the actual range to eliminate uncertainties.
It is harder to comprehend relative uncertainty. Relative uncertainty can be described as an estimate of the range that is within the entire range of the targeted value. It occurs whenever the estimate is larger than the measurement with the highest value that was taken or when the target value is greater than the highest measurement taken. This is also referred to as risk-based on statistical analysis. If, for instance, there’s a 20% probability that the merchandise under test will provide an absolute percentage of 100 percent then there’s a twenty percent chance that the product could yield an absolute percentage of zero.
To calculate the uncertainties associated with measurements using the normal distribution, it is utilized. It is the distribution of true values within a given range. It is visualized as the bell-shaped curve. The uncertainty range represents its length and the standard deviation is the width.
Standard deviations are a measurement of the error created in any given data item. The spread, also known as the range-effect, is the word used to define the variety of values that can be determined based on the shape of the curve. In addition to formulating the standard deviation, the binomial formula could also be employed to determine absolute uncertainty. The binomial equation employs an algorithm called the probability density function defines the degree of uncertainty present in the data set and then calculates the probability of events. Absolute uncertainty refers to the number of times the real value is zero or changes by an amount that is constant.
The primary difference between absolute and relative uncertainty is that the relative error is only a percentage while absolute uncertainty represents the exact value that could be derived through the measurement of the object in question. The confidence intervals typically are the range of values from which the actual value might have been calculated. The relative error can be described as a measurement of expectations rather than actual.



Leave A Comment